Absolute Value

The Absolute Value of a number is its distance from zero (on the number line). Thus, an absolute value is always positive. We indicate absolute value by putting two bars around the number.

Example:

• | 9 | is read as “the absolute value of 9.” Because 9 is 9 spaces from zero on the number line, the absolute value is 9.
• | -4 | is read as “the absolute value of -4.” Because -4 is 4 spaces from zero on the number line, the absolute value is 4.

NEGATIVE DISTANCE MAKES NO SENSE: You can be 50 meters away from your friend, but what would it mean if you were negative 50 meters away from your friend? That would be meaningless. Sure, you might be 50 meters to the other side of your friend, but you’re still 50 meters away. Think about absolute value the same way.

The most important thing to remember about absolute value is the concept of OR. Whenever you’re dealing with absolute value problems, remember that the stuff inside the absolute value brackets does not change

The most important thing to remember about absolute value is the concept of OR.
Whenever you’re dealing with absolute value problems, remember that the stuff inside the absolute value brackets does not change - the stuff on the other side of the equal sign does!!!

In other words: | X - 8 | = 4
Means that: X - 8 = 4 OR X - 8 = -4

Notice there’s no need to do anything to “X - 8?”

Do NOT touch anything inside the absolute value brackets! Never, ever, ever touch anything inside the absolute value brackets.

Instead, follow these steps:

• Get the absolute value brackets by themselves on one side of the = sign.
• Set up the “or” proposition. In other words, once you have an absolute value set up, you have two possibilities:
• Check to see whether each solution is valid by putting each one back into the original equation and verifying that the two sides of the equation are in fact equal.

So long as you remember that there are always two solutions to any absolute value problem (there’s always an “or”) and that you change the non-bracketed side of the equation when converting from absolute value into two distinct equations, you’ll be all set!

Example: Solve for w, given that 12 + | w - 4 | = 30.

STEP 1: Isolate the expression within the absolute value brackets: 12 + | w - 4 | = 30 => | w - 4 | = 18
STEP 2: Once you have an equation of the form | x | = a & a > 0, you know that x = a. Remove the absolute value brackets and solve the equation for 2 different cases
STEP 3: Check to see whether each solution is valid by putting each one back into the original equation and verifying that the two sides of the equation are equal.

- In CASE 1, the solution, w = 22, is valid because 12 + | 22 - 4 | = 12 + 18 = 30.
- In CASE 2, the solution, w = -14, is valid because 12 + | -14 - 4 | = 12 + 18 = 30.

Solved Example 1 
Solve for n, given that | n + 9 | - 3n
A. {-3}
B. {3}
C. {-3,3}
D. {0}

Solution: Isolate the expression within the absolute value brackets and consider both cases.

Step 1: | n + 9 | = 3 + 3n
Step 2:
CASE 1: N + 9 IS POSITIVE
n + 9 = 3 + 3n
n = 3
CASE 2: N + 9 IS NEGATIVE
n + 9 = - (3 + 3n)
n = -3

Step 3: The first solution, n = 3, is valid because | (3) + 9 | - 3 (3) = 12 - 9 = 3.
However, the second solution, n = -3, is not valid because | n + 9 | = 3 + 3n does not hold true when n = -3.

Lets check:
LHS: | n + 9 | = | (-3) + 9 | = 6
RHS: 3 + 3n = 3 + 3 (-3) = - 6

Therefore, the sides are not equal and therefore n = -3 is not a solution. It's imperative we perform the third step for all absolute value questions.

The correct answer is B.

Inequalities and Absolute Value

This is where most of our AP Guru students really seem to go bananas. Many students have a total freak out when they see something like this:
| X - 3 | > 6

To understand the concept, it is helpful to try to visualize the problem with a number line.

For a simple equation such as | x | = 5, the graph of the solutions looks like this:

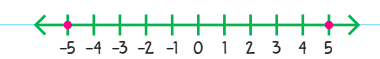
When absolute value is used in an inequality, the unknown generally has more than two possible solutions. Indeed, for a simple inequality such as | x | < 5, a simple way understand this inequality is to say “x must be less than 5 units from zero on the number line.”

Absolute values can often more difficult to graph than the one above. Consider, for instance, the inequality | x + 2 | < 5. This seems more difficult, because the “ + 2 ” term seems to throw a wrench into our distance-on-the-number-line interpretation of this problem.

However, there is a relatively straightforward way to think about this problem. First, create a number line for the term inside the absolute value bars. Here, we create a number line for “x + 2.” We can see that this expression as a whole must be between - 5: and 5:

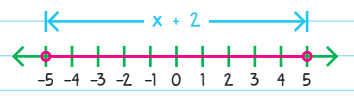
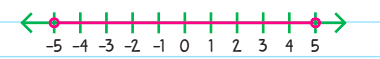
In other words, x + 2 must be less than 5 units away from zero on the number line. We cannot stop there - we must graph for x alone. How does the “ + 2 ” change our graph? It forces us to shift the entire graph by 2, because the absolute value expression will be equal to zero when x = - 2. Thus, the graph for x alone will look like this:

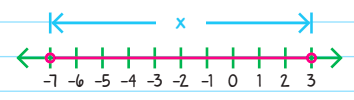
Notice that the center point for the possible values of X is now - 2, which is the value for x that fits X + 2 = 0. This is the “center point” for the number line graph. The distance from the center point (-2) to either end point remains the same.

From this example, we can extract a standard formula for interpreting absolute value. When | x + b | = c, the center point of our graph is -b The equation tells us that x must be exactly c units away from -b. Similarly, for the inequality | x + b | < c, the center point of the graph is -b , and the “less than” symbol tells us that x must be less than c units away from -b.

Example: What is the graph of | x - 4 | < 3?

Solution: Based on this formula, the center point of the graph is -(-4) = 4, and x must be less than 3 units away from that point:

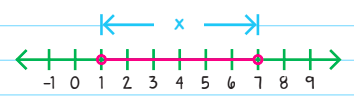
We can also solve these types of problems algebraically. Recall that equations involving absolute value require you to consider two scenarios:

1. One where the expression inside the absolute value brackets is positive
2. Where the expression is negative

The same is true for inequalities. However, remember the ONE key twist: the sign is reversed for the negative possibility!

In other words, to convert this into an “or” propositions: | X-3 | > 6

You get: X - 3 > 6 OR X - 3 < -6

Why do you reverse the sign when you do this? Rather than explain it verbally, let’s look at it mathematically. Let’s say you have this: | X | > 3

It means that “the distance of x from 0 on the number line is greater than 3.” That’s the precise definition of absolute value and of this inequality.

But what if you do this: X > 3 OR X > -3

That makes no real sense. So, x is greater than 3, but also greater than 3? The second piece of information doesn’t help at all...it’s useless. It’s like telling someone you’re taller than six feet but also taller than 5’9”.

But if we remember to switch the sign, we get: X > 3 OR X < -3

That makes way more sense! X is either greater than 3 or less than -3, meaning that its distance from 0 on the number line will remain larger than 3!

So long as you understand the concept of flipping the sign when given the negative “OR” possibility, you understand the hardest stuff you’ll ever be asked about absolute values on this exam.

To test your understanding, solve the following equation: 2 | X - 5 | + 3 > 19

Solving the equation, we should get:

2 | X - 5 | > 16
| X - 5 | > 8

Now we set up our “OR's,” remembering to flip the sign at the right time:
X - 5 > 8 OR X - 5 < -8
X > 13 OR X < -3

Let’s try this one more time: Solve 3 | 2X + 4 | < 3X + 9
We get: | 2X + 4 | < X + 3

Next, I set up my “OR's”:
2X + 4 < X + 3 OR 2X + 4 > -X - 3
X + 4 < 3 OR 3X + 4 > -3
X < -1 OR X > -(7/3)

Therefore, X is less than -1 or greater than -(7/3). Done! X can be anything that isn’t between -1 and -(7/3).

Complex Absolute Value Equations

So far, we have only looked at absolute value equations that have one unknown inside one absolute value expression. However, these equations can get more complicated by including more than one absolute value expression. The equation contains one variable and at least one constant in more than one absolute value expression.

Example: If | x - 2 | = | 2x - 3 |, what are the possible values for x?

Because there are two absolute value expressions, each of which yields two algebraic cases, it seems that we need to test four cases overall:

1. The positive/positive case: (x -2) = (2x - 3)
2. The positive/negative case: (x -2) = - (2x - 3)
3. The negative/positive case: - (x -2) = (2x - 3)
4. The negative/negative case: - (x -2) = - (2x - 3)

However, note that case 1 and case 4 yield the same equation. Likewise, case 2 and case 3 yield the same equation. Thus, you only need to consider two real cases: one in which neither expression changes sign, and another in which one expression changes sign.

CASE A: Same Sign
(x - 2) = (2x - 3)
x = 1

CASE B: Different Signs
(x - 2) = - (2x - 3)
x = 5/3

You also have to check the validity of the solutions once you have solved the equations.
Both solutions are valid, because

|1 - 2 | = |2(1) - 3 | = 1, &
| 5/3 - 2 | = |2(5/3) - 3 | = 1/3